YOU ARE LEARNING:
The Quadratic Formula

The Quadratic Formula
The quadratic formula gives us a method of solving any quadratic equation to a high degree of accuracy.
Another way to solve quadratic equations which do not factorise is to use the quadratic formula.
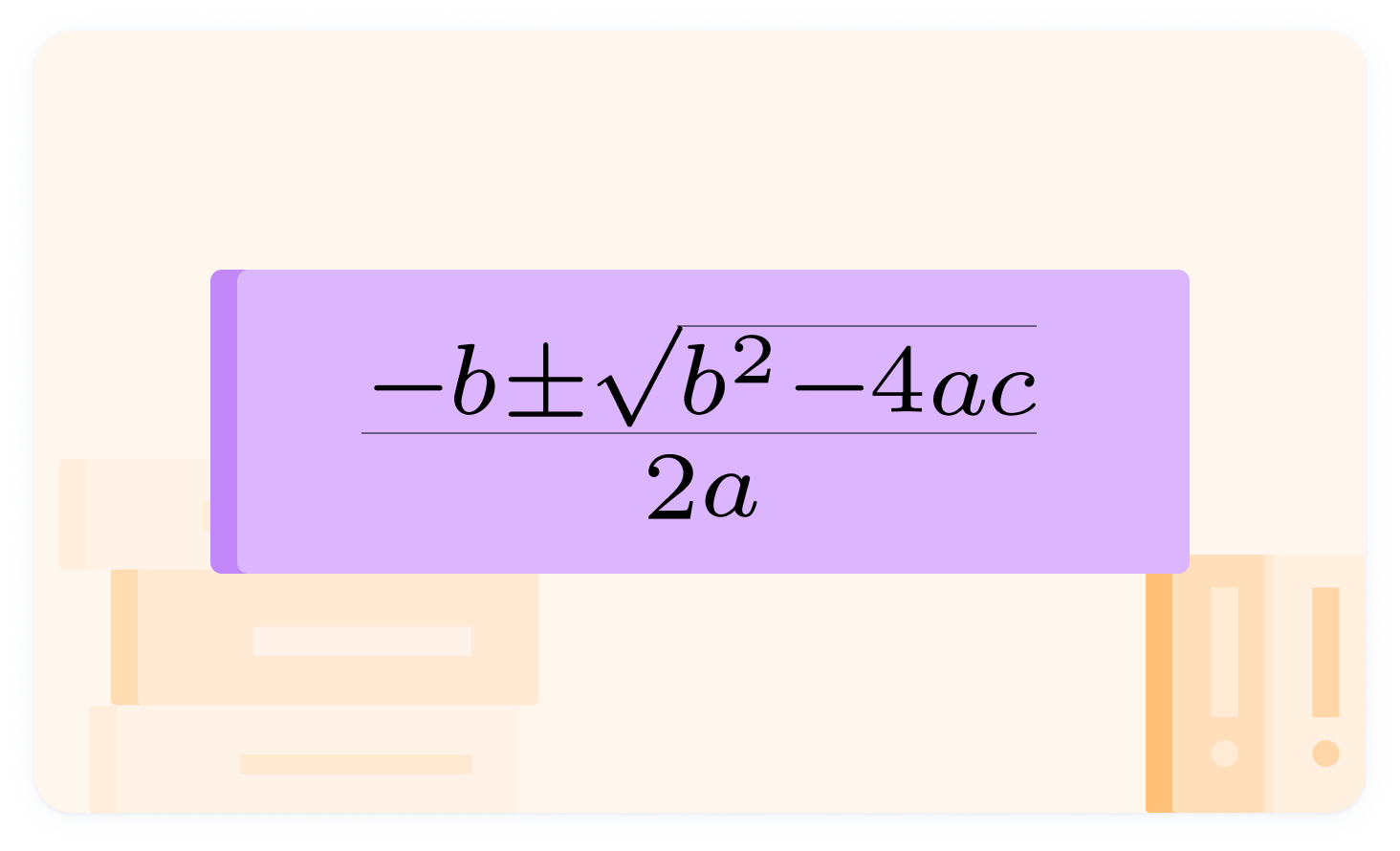
This is the quadratic formula
It is used for equations of the form: ax2+bx+c=0 where a,b and c are known values.
When might you use the Quadratic Formula to solve an equation?

Let's have a go! Find the values of x for 3x2+11x+6=0
Remember x=2a(−b±b2−4ac) for quadratics in the form ax2+bx+c.
What is the value of a?

What is the value of b?

What is the value of c?

Put these values into the formula
x=2×3(−11±112−4×3×6)
Simplify it
x=6(−11±49)
Remember there are 2 equations here
x=6(−11+49) and x=6(−11−49)
We can solve each equation separately
x=6(−11+49) can be simplified to x=6−4=3−2
If x=6(−11−49), what is x?

Nice work! Now use the quadratic formula to solve 3x2+6x+1=0

Use the quadratic formula to solve 6x2+7x+2=0

