Forces in Motion

YOU ARE LEARNING:

Vector Diagrams: Resultant Force

In some cases we need to find the resultant force where forces act in different directions, and represent this in vector diagrams.

In most situations there is more than one force acting upon an object. We can replace the individual forces acting with a single force. What do we call this force?

What effect does the resultant force have?

Two people are pushing a box and applying a force. Are the forces parallel, opposite or perpendicular in direction?

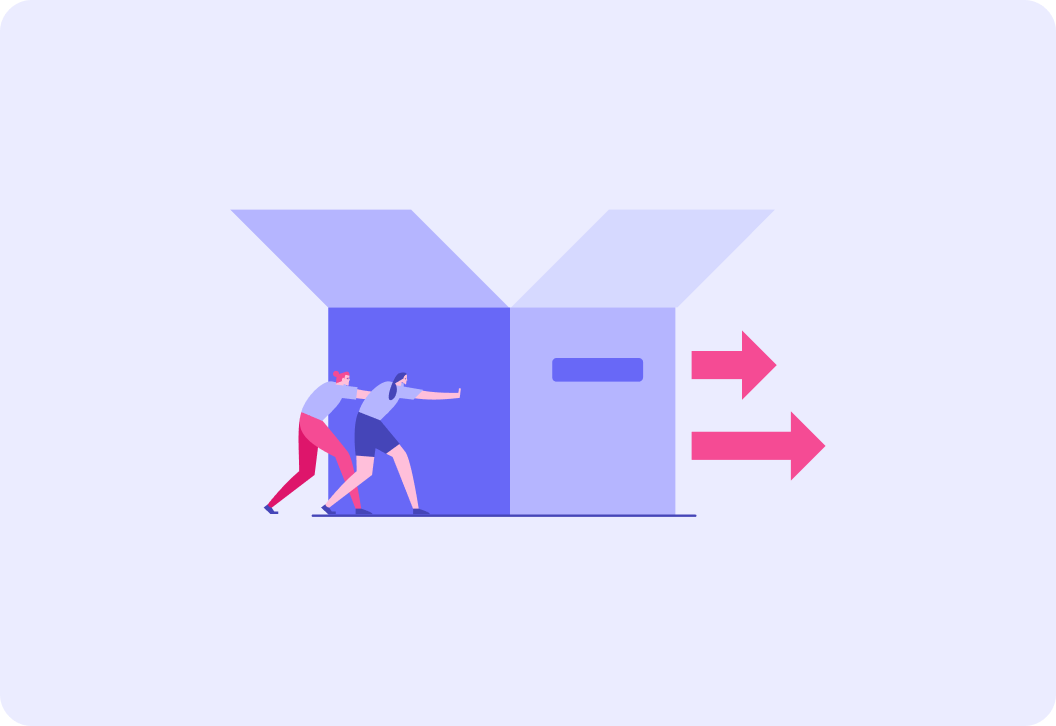
Both forces are acting in the same direction. To find the magnitude of the resultant force would you add or subtract the forces?

Two forces are acting on a box. One force is applied from the person pushing the box, and the opposing force is caused by friction.

True or false? The forces are parallel and opposite in direction.

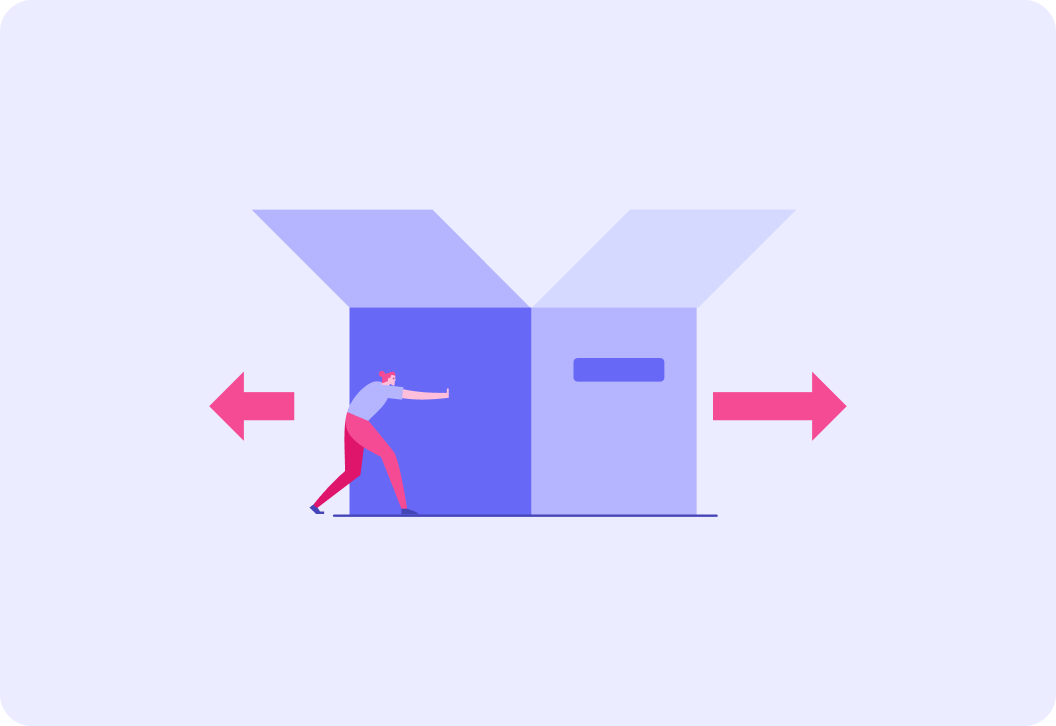
To find the magnitude of the resultant force, would you add or subtract the forces?

In some situations we need to find the resultant force when the individual forces are not parallel. To do this, we must first draw a scaled diagram of all the individual forces.

A force always has both magnitude and direction, so you also must state the direction of a resultant force. To find the direction, you should measure the angle with a protractor, and state the angle as a bearing . A bearing is typically written as a 3 digit number, for example $45\degree$ would be written as $045\degree$ .

A resultant force acts in a south easterly direction. How might you write the direction of force as a bearing?