Number

YOU ARE LEARNING:

Surds: Adding and Subtracting

Surds: Adding and Subtracting

We can also add and subtract surds together, which can be a little more complicated.

In order to add or subtract surds, they need to have the same root. This means that the number inside the square root signs must be the same.

Can you add these surds together as they are?

$\sqrt{10}+\sqrt{6}$

Adding surds means that you can have multiples of that surd:

$\sqrt8 +\sqrt8=2\sqrt8$

Similarly:

$2\sqrt8+ 6\sqrt8=8\sqrt8$

Let's find:

$3\sqrt{4}+12\sqrt{4}$

Are they the same root? Yes or no

They're the same, so we can add them

To add them, all we need to do is add together the numbers before the square root.

What is $3+12$ ?

Nice! We've added them together

The final answer is $15\sqrt{4}$ .

What is $6\sqrt{2} + 3\sqrt{2}$ ?

Unfortunately, surds don't always have the same number in their root.

In order to add surds with different numbers inside the roots, we can first look to simplify them. This leads to a smaller number inside the root:

$\sqrt{10} \rightarrow5\sqrt2$

For example, write $\sqrt8 + \sqrt{32}$ in terms of $\sqrt2$

We can simplify $\sqrt{8}$

To do this, we need to find two factors: one surd and one perfect square.

$4$ and $2$ multiply to make $8$

$4$ is also a perfect square. So, we can simplify $\sqrt{8}$ to $2\sqrt{2}$

We can also simplify $\sqrt{32}$

To do this, we follow the same process as we just did with $\sqrt{8}$ .

Write $32$ as a product of a square and $2$

Express these as different surds

$\sqrt{32}=\sqrt{16 \times 2}=\sqrt{16} \times \sqrt{2}$

What is $\sqrt{16}$ ?

We're left with $4 \times \sqrt{2}$

$\sqrt2\times 4=4\sqrt2$ . So, $\sqrt{32}=4\sqrt2$

Add our new surds together

$\sqrt{32}+\sqrt8$ is the same as $4\sqrt{2}+2\sqrt{2}$ .

What is $4\sqrt{2}+2\sqrt{2}$ ?

The final answer is $6\sqrt{2}$

Good work! We've added two surds with different root numbers 😃

What is $\sqrt{27} +\sqrt{12}$ in terms of $\sqrt3$

Give $\sqrt{20} +\sqrt{80}$ in terms of $\sqrt{5}$

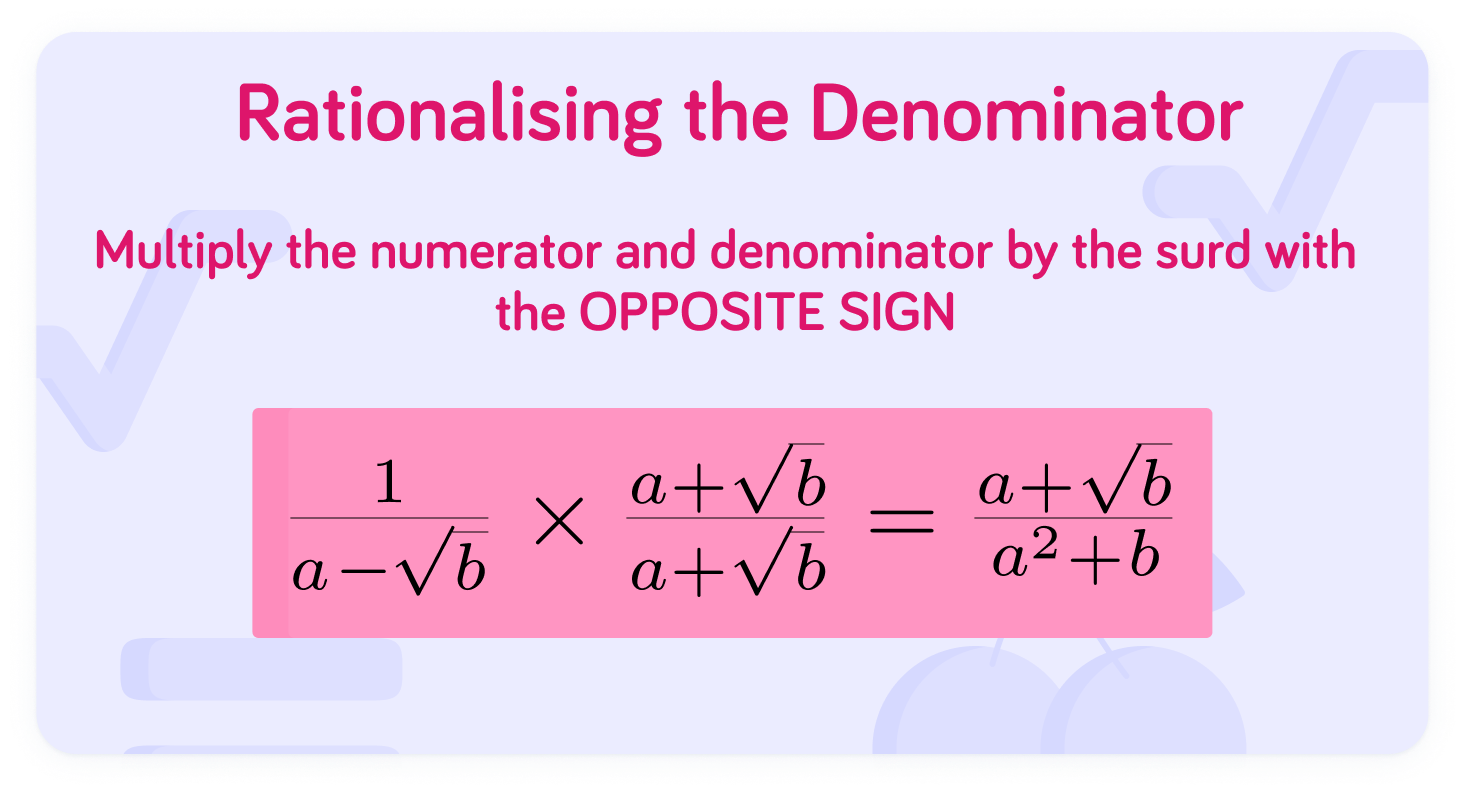
This means that when we expand the brackets, there will be a $+\sqrt5$ and a $-\sqrt5$ which cancel out, leaving us with a rational denominator.

Rationalise $\dfrac{1}{2+\sqrt{5}}$

Surds are an exact way to write irrational numbers.

Let's have a go at solving this one! $\dfrac{4}{\sqrt5}$

Multiply the top by the surd

$\dfrac{4}{\sqrt5} \times \dfrac{\sqrt5}{\sqrt5}=\dfrac{4\sqrt5}{\sqrt5 \times \sqrt5}$

Multiply the bottom together

$\dfrac{4\sqrt5}{\sqrt5 \times \sqrt5}=\dfrac{4\sqrt5}{5}$

Nice! 👌

$\dfrac{4}{\sqrt5}$ can be rationalised to $\dfrac{4\sqrt5}{5}$

If the fraction looks like: $\dfrac{3}{1-\sqrt{5}}$

We need to multiply by: $\dfrac{1+\sqrt{5}}{1+\sqrt{5}}$

Rationalising the denominator

Multiply the numerator and the denominator by the surd with the OPPOSITE sign

Rationalise $\dfrac{1}{1+\sqrt{2}}$

Now let's try solving $\dfrac{3}{1-\sqrt{5}}$

Multiply by the denominator with the opposite sign

$\dfrac{3}{1-\sqrt{5}} \times \dfrac{1+\sqrt{5}}{1+\sqrt{5}}$

Multiply the numerators

$\dfrac{3}{1-\sqrt{5}} \times \dfrac{1+\sqrt{5}}{1+\sqrt{5}}=\dfrac{3+3\sqrt5}{(1-\sqrt{5}) \times (1+\sqrt{5})}$

Multiply the denominators

$\dfrac{3+3\sqrt5}{(1-\sqrt{5}) \times (1+\sqrt{5})}=\dfrac{3+3\sqrt5}{-4}$

Make the denominator positive

$\dfrac{3+3\sqrt5}{-4} \times \dfrac{-1}{-1}=\dfrac{-3-3\sqrt5}{4}$

Great work! 💪

This can't be simplified any more, so the answer is $\dfrac{-3-3\sqrt5}{4}$

Multiply the numerator and the denominator by the surd

This is a key step!

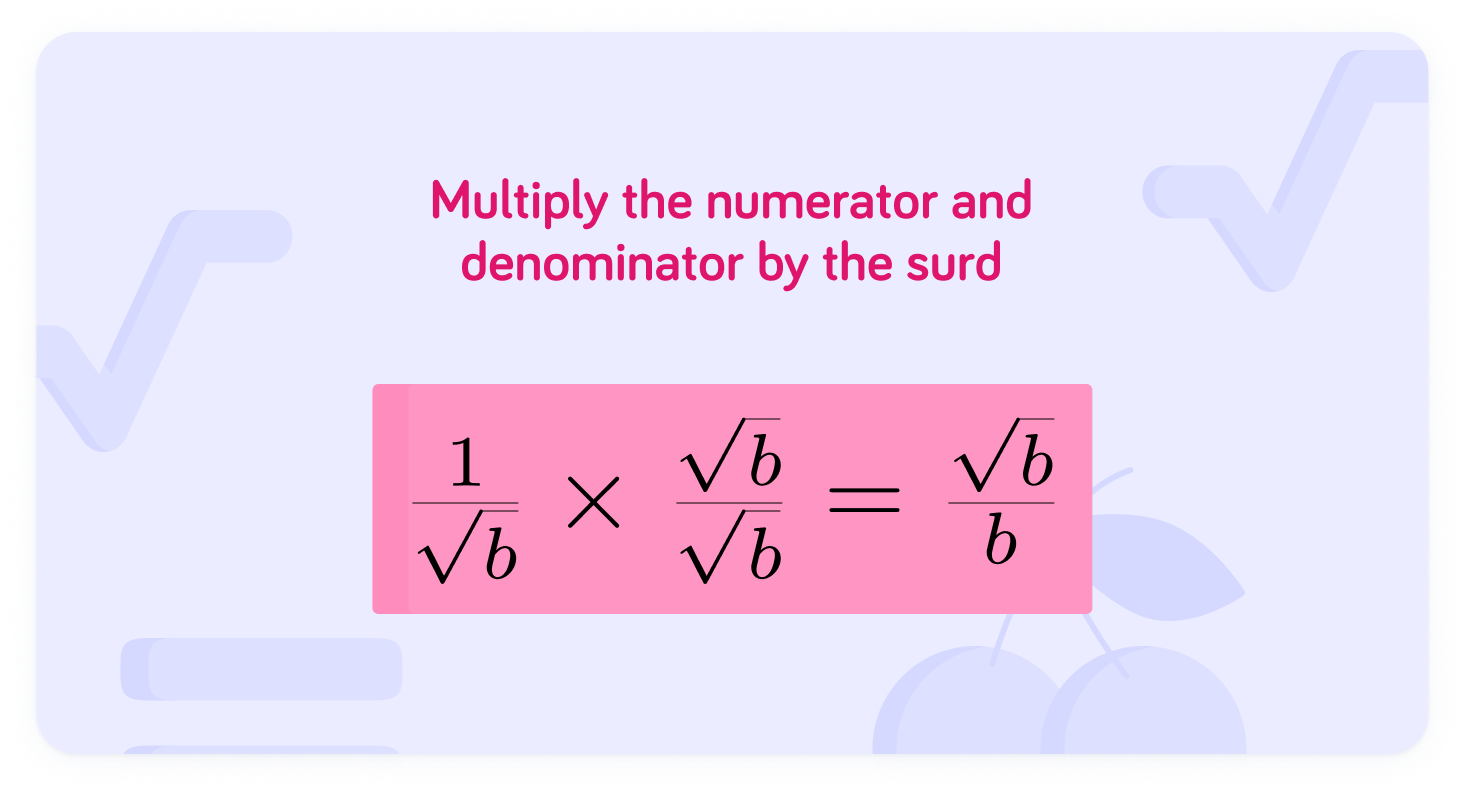
Rationalise $\dfrac{1}{\sqrt{13}}$

For a fraction that looks like $\dfrac{4}{\sqrt5}$ , we need to multiply by $\dfrac{\sqrt5}{\sqrt5}$ to make the denominator an exact number, as a square root multiplied by itself is a whole number.