YOU ARE LEARNING:
Surds: Multiplying and Dividing

Surds: Multiplying and Dividing
We can multiply and divide surds together, to form new surds.
We can calculate with surds, subject to a few rules.
To multiply, multiply the numbers in the root
a×b=a×b
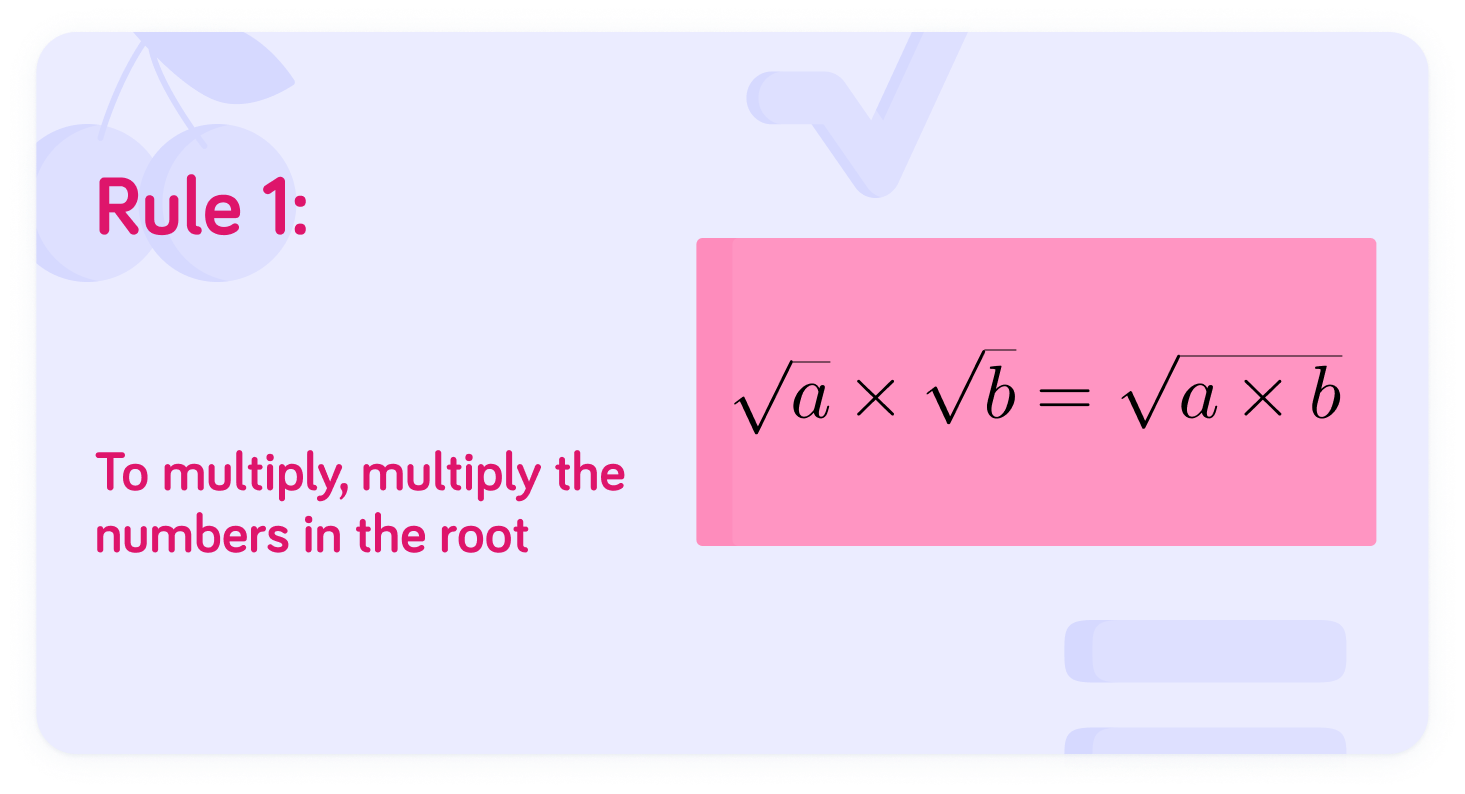
So:
3×6=3×6=18
What is 2×5?

What is 12×5 as a single square root?

To divide, divide the numbers in the root
ba=ba
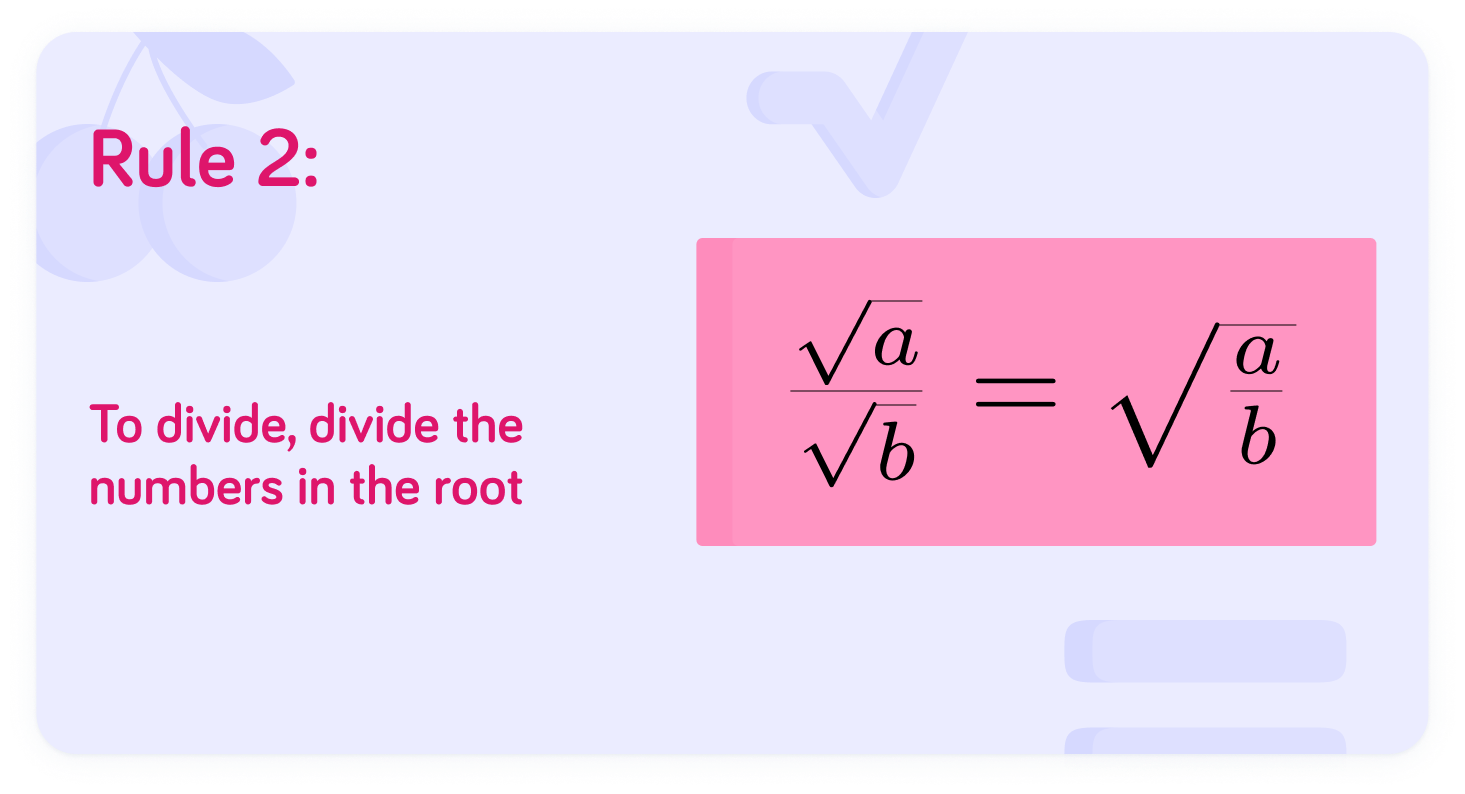
Therefore:
26=26=3
What is 1545 as a single square root?

We can also multiply and divide mixed surds, like those below, by operating on the number and surd parts separately.
35×76
Let's find:
35×76
Multiply the numbers together: 3×7

Now multiply the surds 5×6

Put these two parts together
The final answer is 2130. This can't be simplified, as 30 does not have a factor that is a square number.
What is 2410÷85?

Find 35×43

Let's try a division:
164÷42
Treat is as two separate divisions
16÷4 and 4÷2.
Find 16÷4

Find 4÷2

Put these two parts together
The final answer is 42.
