Graphs

YOU ARE LEARNING:

Straight Line Graphs 3

We can identify the gradient and y-intercept if we are given a set of coordinates which feature on a line.

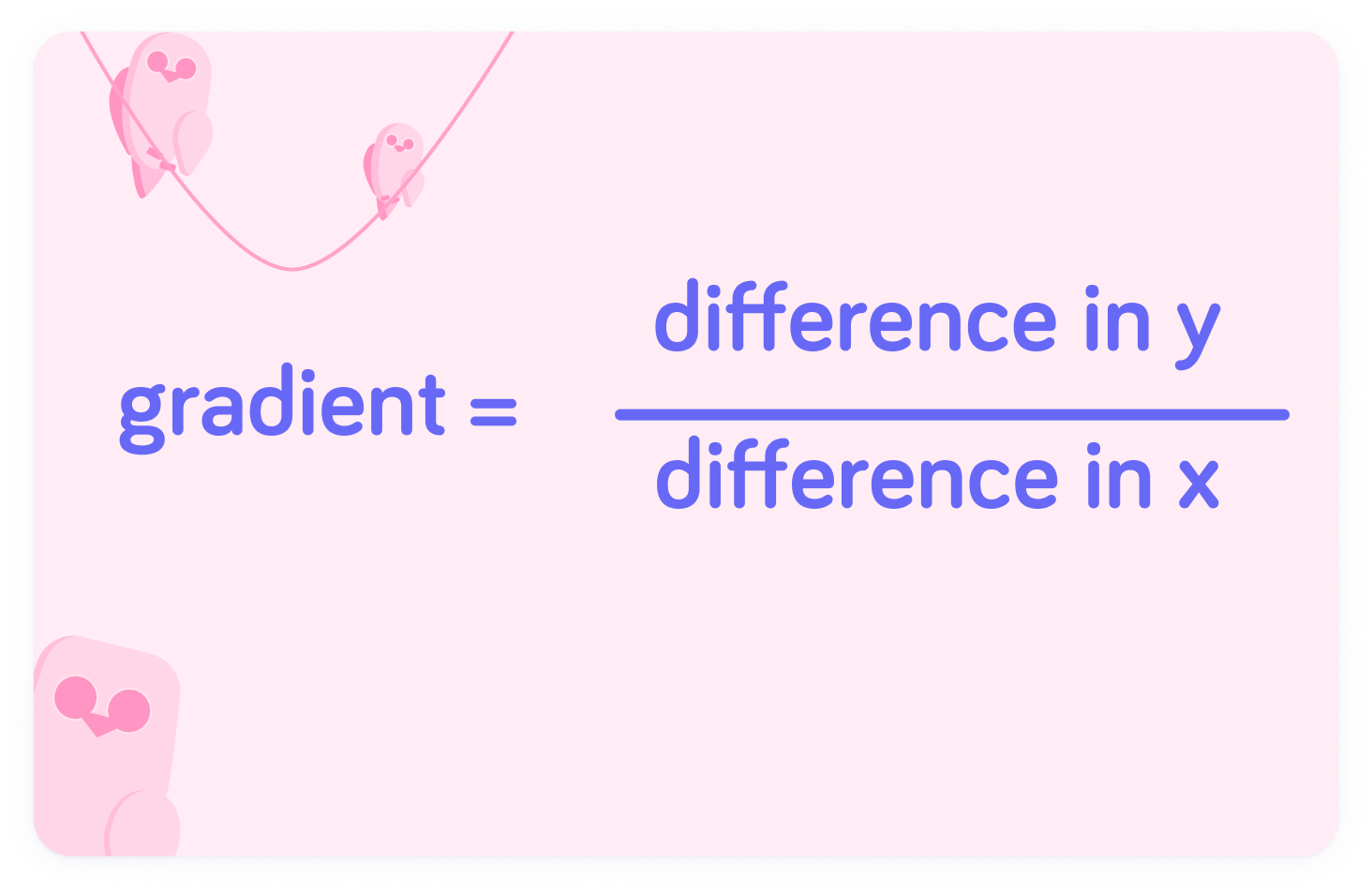
The gradient can be found by using a formula with coordinates located on a line.

Find the gradient of the line joining $(3,4)$ and $(5, 6)$

Let's find the equation of a line when we do not know the y-intercept.

For example, let's find the equation of the line joining $(6,20)$ and $(4,14)$

We can't immediately see the y-intercept

Neither of the points have an $x$ value of 0, so neither of them are the point at which the line crosses the y-axis.

Start by finding the gradient

Remember the formula: $\dfrac{difference \space in\space y}{difference \space in \space x}$

What is the gradient between these points?

Substitute the gradient into the equation

Now we have a value for $m$ , we can put this into an equation containing one of the points. Remember, the coordinates contain the x and y values at that point.

Let's do this for the point $(4,14)$

For $y=mx+c$ , $14=3(4)+{\color{#21affb}c}$ . We can rearrange this to find $c$ .

Subtract 12 from both sides

$14-12={\cancel{12}}+ c \space \rightarrow\space c=2$

Nice! 😎

Now we know $m$ and $c$ , we can see that our equation is $y=3x+2$ . You can check this by substituting in the values of the other point.