Number

YOU ARE LEARNING:

Indices: Fractional Powers

Indices: Fractional Powers

Fractional indices are like finding roots, while negative indices are like finding reciprocals. We can also combine these in more difficult scenarios.

The power in an index number is not always an integer.

$x^{\frac{1}{2}}$ is an example of an index number with a fractional power.

To understand how fractional powers work, let's consider $x^{\frac{1}{2}}$ and multiply it by itself. What is $x^{\frac{1}{2}}\times x^{\frac{1}{2}}$ ?

When we multiply a number by itself, we get a square, for example $a\times a=a^2$ . In this example what is $a$ ?

In our example, we had $x^{\frac{1}{2}}\times x^{\frac{1}{2}}=x$ .

This means that $x^{\frac{1}{2}}$ is the square root of $x$ or $x^{\frac{1}{2}}=\sqrt{x}$ .

Let's have a look at the index number with fractional power $\frac{1}{3}$

Our example is $y^{\frac{1}{3}}$ .

This time, work out $y^{\frac{1}{3}} \times y^{\frac{1}{3}}\times y^{\frac{1}{3}}$ .

What is a number that is multiplied by itself three times, for example $a\times a\times a$ ?

We know that $y^{\frac{1}{3}} \times y^{\frac{1}{3}}\times y^{\frac{1}{3}}=y$

This means that $y^{\frac{1}{3}}$ is the cube root of $y$ or $y^{\frac{1}{3}}=\sqrt[3]{y}$ .

We can see a pattern here $x^{\frac{1}{2}}=\sqrt{x}$

$y^{\frac{1}{3}}=\sqrt[3]{y}$ .

The general rule, a fractional power with numerator $1$ , is the root of the base number of the same value as the denominator.

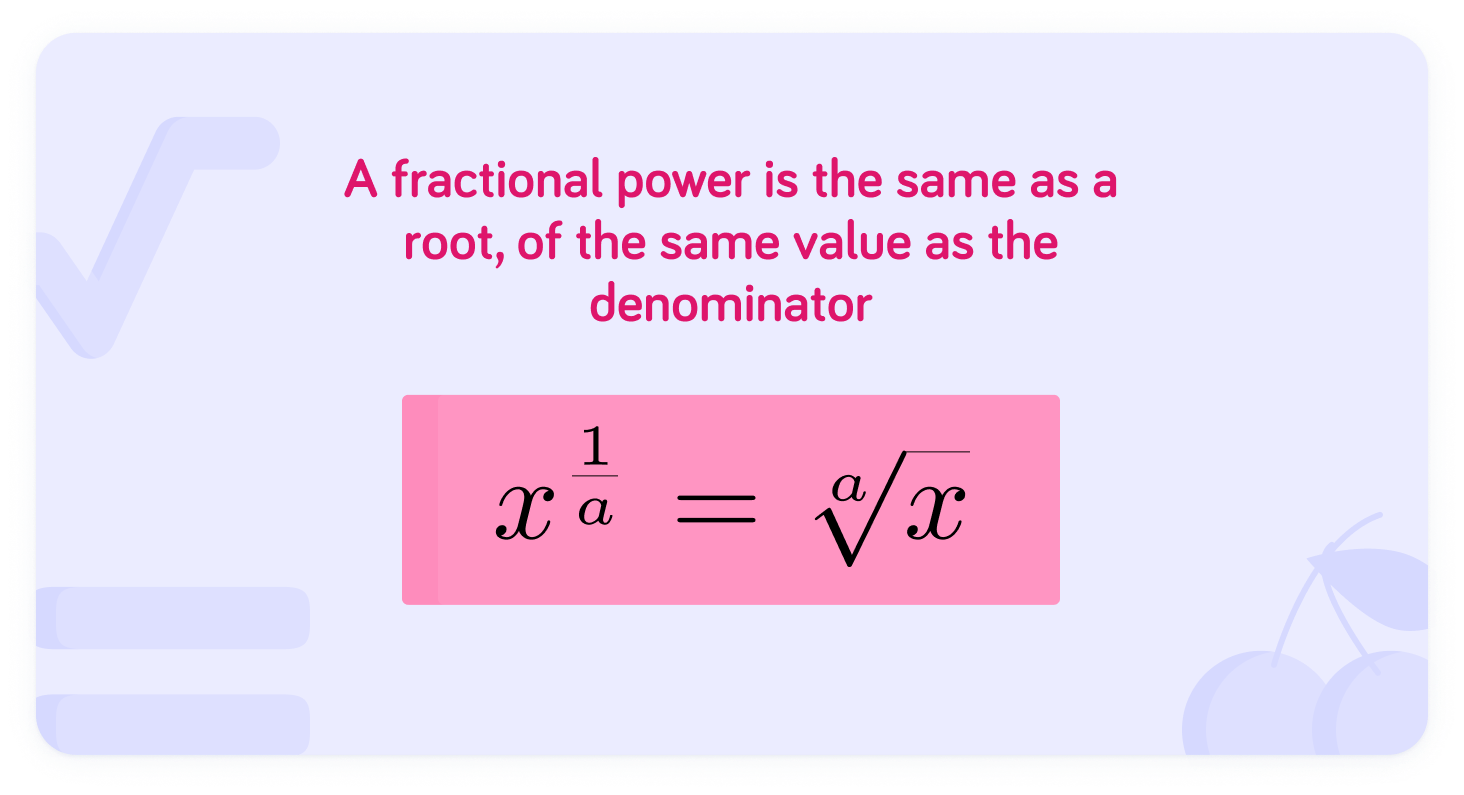
What is the meaning of $a^{\frac{1}{6}}$ ?

We can work out some values. What is $16^{\frac{1}{2}}$ ?

Let's try another one, what is $27^{\frac{1}{3}}$ ?

We can apply this to other fractional powers:

$64^\frac{1}{3}=\sqrt[3]{64}=4$

$625^{\frac{1}{4}}=\sqrt[4]{625}=5$

What is $32^{\frac{1}{5}}$ ?

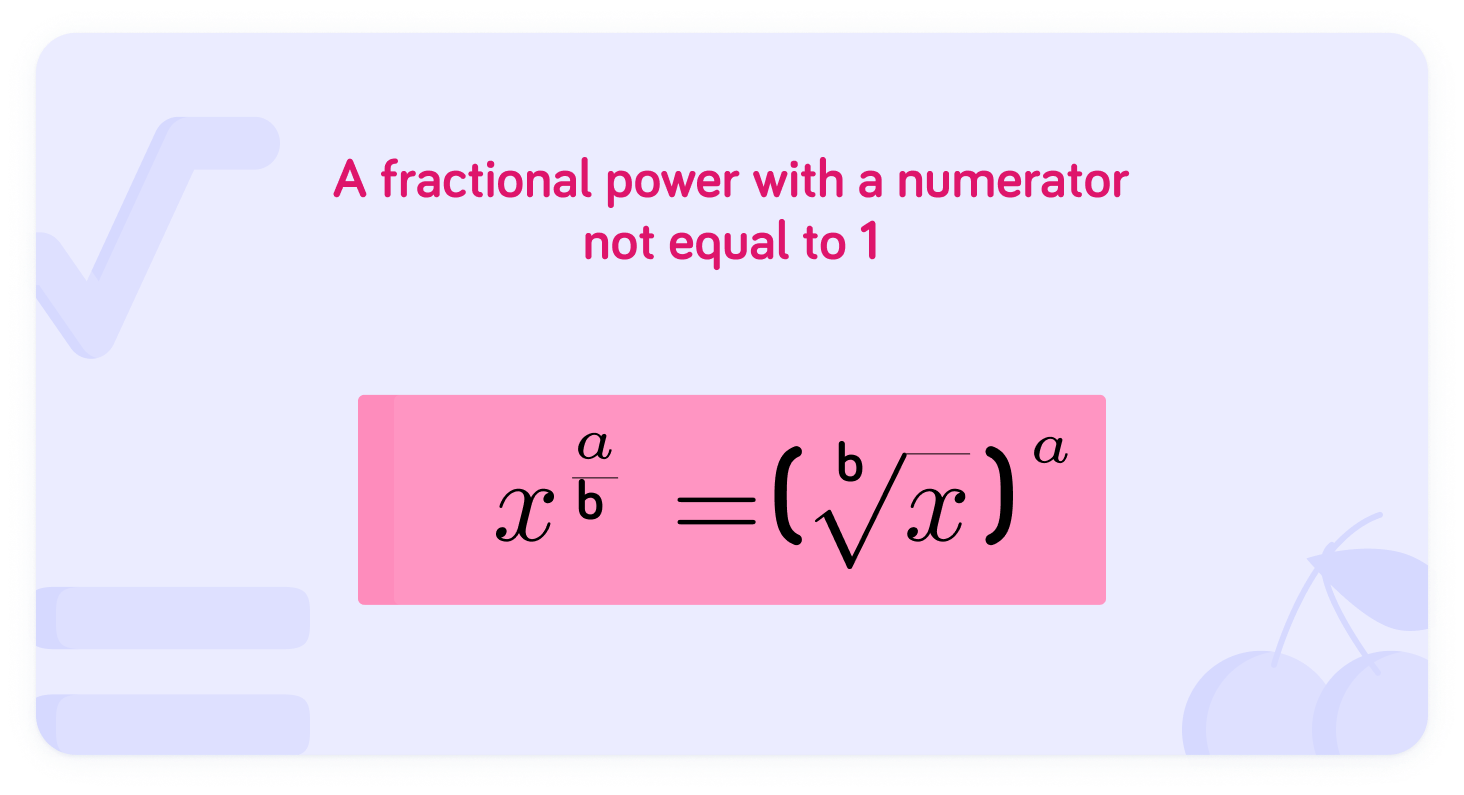
We can also use larger fractional powers, where the numerator is more than one.

Let's try an example: $8^{\frac{2}{3}}$

We can split the fractional power $\frac{2}{3}$ into two parts as $\frac{2}{3} = \frac{1}{3} \times 2$ . How can we show this using brackets?

We can now work out the answer is two parts. First, what is the result of $8^{\frac{1}{3}}$ ?

Replace $8^{\frac{1}{3}}$ with $2$ in $(8^{\frac{1}{3}})^2$ .

$(2)^2$

Find $(2)^2$ .

Nice! 👍

The answer is $8^{\frac{2}{3}}=4$ .

Let's try a slightly harder one. What is $64^{-\frac{2}{3}}$ ?

We can generalise the rule

Notice that there is a negative in the power too, but we can use the rule in the same way.

The power in $64^{-\frac{2}{3}}$ is $\frac{-2}{3}$ , how can we split this down to use in the rule?

We now have $64^{-\frac{2}{3}}=(64^{\frac{1}{3}})^{-2}$ . Let's work out what is in the brackets, what is $64^{\frac{1}{3}}$ ?

We can now replace $64^{\frac{1}{3}}$ in $(64^{\frac{1}{3}})^{-2}$ with $4$ .

This gives $(4)^{-2}$ .

Finally we work out $(4)^{-2}$ . Take care with the negative sign - this is asking you to take the reciprocal of the positive power.

You have shown that $64^{-\frac{2}{3}}=\dfrac{1}{16}$

Well done! 👍

Final question: what is the value of $27^\frac{2}{3}$ ?

Summary!

A fractional power with a numerator of $1$ is the same as the root of the same value as the denominator

$x^{\frac{1}{2}}=\sqrt{x}$

$y^{\frac{1}{3}}=\sqrt[3]{y}$

A fractional power with a numerator greater than $1$

is the root of the base number of the same value as the denominator then raised to the power of the same value as the numerator.