Number

YOU ARE LEARNING:

Indices: Multiplying and Dividing

We can carry out multiplication and division of indices, giving the results in terms of powers.

Numbers like $4^5$ and $x^y$ are called indices.

Indices have base numbers and powers.

In $4^5$ which number is the base number?

In $4^5$ , the base number is $4$ and the power is $5$ .

$4^5=4\times 4\times 4\times 4\times 4$

The same principle applies for algebraic expression. Which is the base number in $x^y$ ?

In the term $x^y$

$x$ is the base number $y$ is the power - this is also sometimes called the index.

$x^{3}\times x^{5}$

Let's look at multiplying two indices together.

$x^{3}\times x^{5}$

$x^3$ multiplies $x$ by itself 3 times. How else can this be written?

Similarly, $x^5$ multiplies $x$ by itself 5 times

This can be written $x \times x \times x \times x \times x$

We can now multiply the two expressions $x \times x \times x$ and $x \times x \times x \times x \times x$ together. What does this give us?

We now have $x\times x\times x\times x\times x\times x\times x\times x$ or $x$ multiplied by itself eight times. What is another way of writing this?

We have found our answer!

$x^3\times x^5=x^8$

What do you notice about the powers in the multiplication $x^3\times x^5=x^8$ ? What did we do to the powers?

This is our first rule!

When multiplying numbers raised to indices, we add the powers.

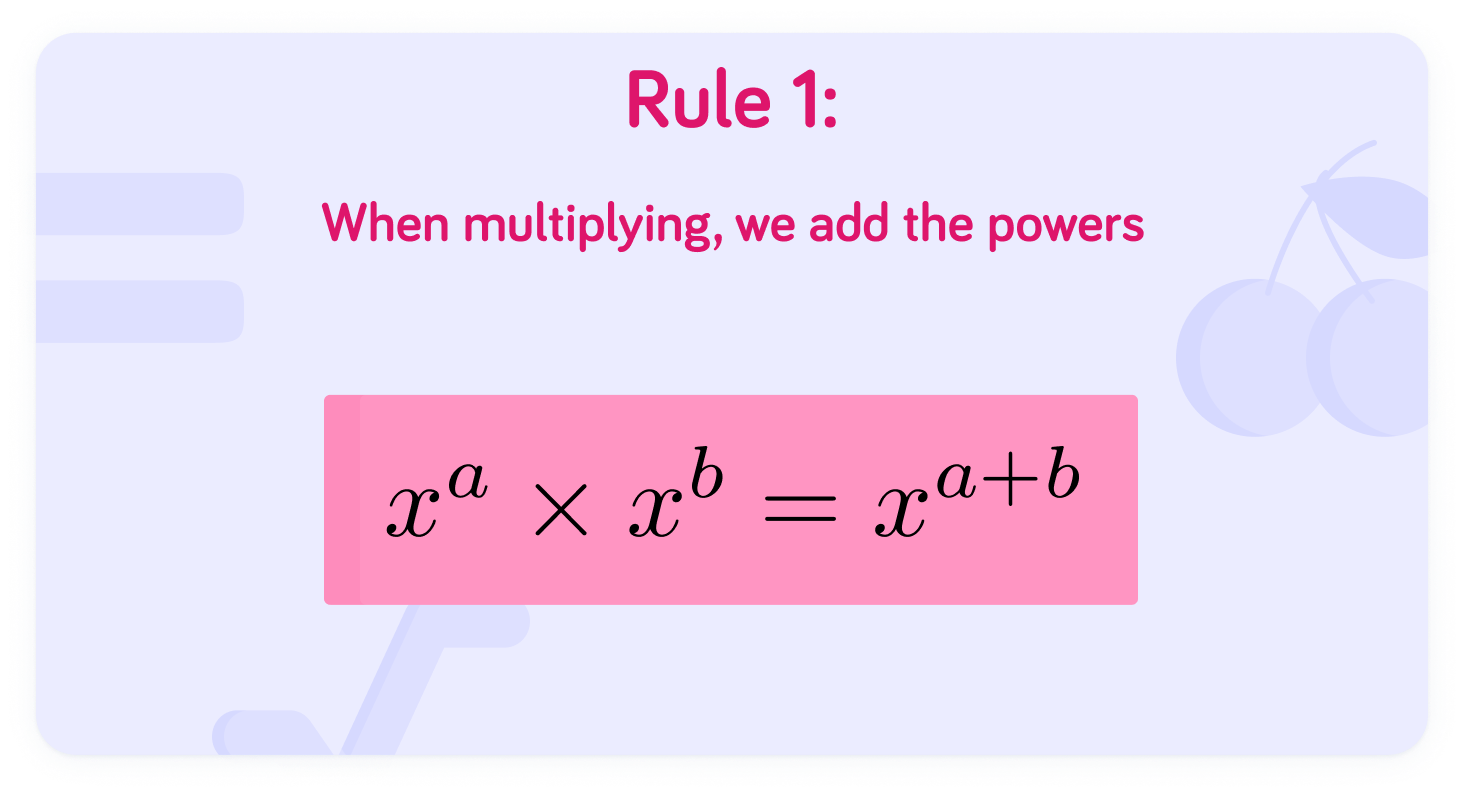
Let's try $4^5\times 4^6$

This is the same as $4^{5+6}$

So what is $4^{5+6}$ as a power of $4$ ?

What is $3^3 \times 3^8$ as a single power of $3$ ?

Let's try something a little more difficult.

What happens when the base numbers are different?

We know that $x^3\times x^5=x^8$ . But what is $x^4\times y^7$ ?

To add the powers, the base number must be the same. How can we simplify $x^2\times y^5\times x^4$ ?

What about dividing indices that have the same base number?

$\dfrac{x^5}{x^3}$

$x^5$ multiplies $x$ by itself 5 times

Therefore, it is the same as $x \times x \times x \times x \times x$

What can $x^3$ also be written as?

This means our expression can be written as:

$\dfrac{x \times x \times x \times x \times x}{x \times x \times x}$

Cancel out pairs of $x's$

$\dfrac{x \times x \times \cancel{x} \times \cancel{x} \times \cancel{x}}{\cancel{x} \times \cancel{x} \times \cancel{x}}$

Simplify $\dfrac{x \times x \times \cancel{x} \times \cancel{x} \times \cancel{x}}{\cancel{x} \times \cancel{x} \times \cancel{x}}$

What do you notice about the powers in the division $\dfrac{x^5}{x^3}=x^2$ ?

This is our second rule!

When dividing two indices with the same base numbers, subtract the powers.

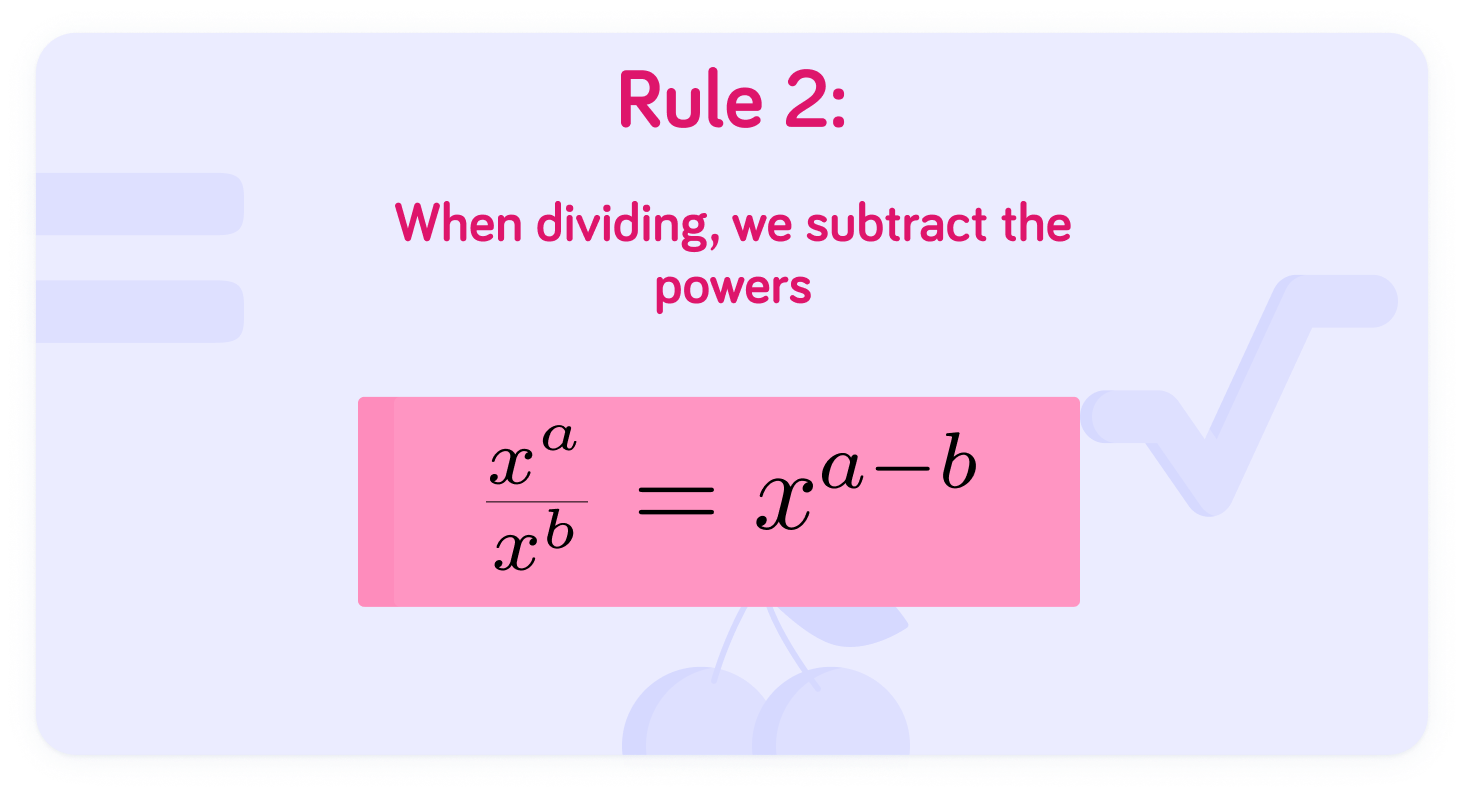
Let's try $7^8\div7^4$

This is the same as $7^{8-4}$

What is $7^{8-4}$ , as a power of 7?

What is $\dfrac{5^{14}}{5^{11}}$ as a single power of $5$ ?

As with multiplication of indices, the rule only works when the base number is the same. How do we simplify $\dfrac{x^7y^5}{x^3}$ ?

We can combine multiplication and division in one expression.

Let's look at $\dfrac{x^2\times x^8}{x^4\times x^3}$ .

Start with the numerator and work out the multiplication

$\dfrac{x^2\times x^8}{x^4\times x^3}=\dfrac{x^{10}}{x^4\times x^3}$

We now have $\dfrac{x^2\times x^8}{x^4\times x^3}=\dfrac{x^{10}}{x^4\times x^3}$ , what is the result of the multiplication in the denominator?

We have now simplified to $\dfrac{x^{10}}{x^7}$ . What is the final answer here?

Summary! When multiplying indices with the same base number

Add the powers $3^5\times 3^7=3^{12}$

When dividing indices with the same base number

Subtract the powers $\dfrac{4^8}{4^5}=4^3$

Both rules only apply when the indices have the same base number.

$x^3\times y^6=x^3y^6$ - we cannot add the powers as the base numbers are different.

We can combine multiplication and division to find our answer.

$\dfrac{x^3\times x^6}{x^4}=\dfrac{x^9}{x^4}=x^5$